 |
Working through the problem with the formulas:
|
|||||||||||||||||||||||||
Dilution Theory / Supplementary Pages:Solutions to the General
|
|
||||
1. One ml of a bacterial culture was pipetted into a 9 ml dilution blank. One-tenth ml of this dilution was pipetted into a 9.9 ml dilution blank. From this dilution, one-tenth ml was plated with 25 ml of culture medium. 220 colonies arose after incubation. How many colony-forming units were present per ml of the original culture?
 |
Working through the problem with the formulas:
|
|||||||||||||||||||||||||
As we understand the so-called "plated dilution" to represent the actual amount of undiluted sample being tested, we can look at the problem (and similar problems) like this: If 220 colonies arose from plating (the equivalent of) 10–4 ml of the culture, then (proportionally) there would have been 220 X 104 (= 2.2 X 106) CFUs per one ml of the original, undiluted culture. This is the reasoning behind the second of the two dilution formulas.
The dilution factor is the inverse of the plated dilution and is meant to inflate the colony count to give the number of CFUs that were in one ml of the original, undiluted sample at the time it was plated out.
Click here for a summary (as presented in a recent Bacteriology 102 lab) of "three ways" by which this problem can be solved.
2. Ten ml of spring water were added to a petri dish to which 40 ml of melted Plate Count Agar were added. After incubation, 35 colonies arose on the plate. What was the count of CFUs per ml of the spring water?
As 35 colonies arose from the 10 ml inoculum, then there had been 3.5 CFUs per ml of the spring water. Note that no formulas are needed for this problem, but if they were to be used, the dilution factor would be 10–1. Why? Remember that the "plated dilution" represents the actual amount of sample being tested which in this case is 10 ml. (In the usual dilution problem – but not in this case – this amount is a small fraction of a gram or ml.) The inverse of the plated dilution – which is the dilution factor – would therefore be 10–1.
3. You are given eight petri dishes of Nutrient Agar and an abundance of pipettes and 9.0 ml dilution blanks, and you need to plate out a sample of milk such that plated dilutions of 10–1, 10–2, 10–3 and 10–4 are achieved in duplicate.
a. Clearly diagram how this may be done with the materials at hand. (As it may take quite awhile for large inocula to soak into plates, do not plate any amount larger than 0.2 ml.)

b. On the plates containing the 10–4 plated dilution, you count 48 colonies on one plate and 54 colonies on the other after incubation. Calculate the number of colony-forming units (CFUs) per one ml of the original milk sample.
| dilution factor | X | no. colonies | = | no. CFUs/ml |
| 104 | X | 51 (the average) | = | 5.1 X 105 |
4. Consider the following dilution scheme:
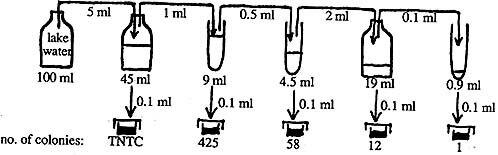
a. Report the total number of CFUs in the entire 100 ml amount of the original lake water sample. (TNTC=too numerous to count.)
| dilutions made | X | amount inoculated | = | "plated dilution" |
| 1/10 X 1/10 X 1/10 | X | 1/10 | = | 1/104 or 10–4 |
|
|
||||
| dilution factor | X | no. colonies | = | no. CFUs/ml |
| 104 | X | 58 | = | 5.8 X 105 |
Multiply CFUs/ml by 100 to get CFUs/100ml. Answer = 5.8 X 107.
b. Would you expect any change in the answer of the above problem if the first dilution was made by adding one ml of sample to 9 ml of diluent? Why or why not?
No. In each case you're making a 1/10 dilution. So the concentration of CFUs per ml of that dilution will be the same.
c. Would you expect the number of viable (living) cells in the original sample to be greater than, less than or the same as the number of CFUs in the sample?
Greater! As a CFU can be one or more cells, there is no one-to-one relationship between the number of colonies seen and the number of original cells. Also, you must consider that there may be viable cells which will not grow under the conditions given (medium, temperature, etc.). So our plate count always gives us a number lower than the real number.
Be sure you know the definitions of cell, CFU and colony as given in the introduction to Experiment 1! Realize that CFU is not strictly a quantitative term. (The cells don't know if their colonies are going to be counted or not!)
d. What dilution is achieved by adding 2 ml of inoculum to 19 ml of diluent? (No, it wasn't a misprint in the diagram.)
It's simply a 2/21 dilution! We get that from 2/(19+2). There is no rule that dilutions have to be 1/10 or 1/100 or something else convenient. As long as we keep track of the math, we can plug any number into our dilution formulas and grind out the number of CFUs per ml or gram.
5. You have obtained a pond water sample and wish to determine the concentration of bacteria which are gram-negative and lactose-fermenting. After making two 1/100 dilutions, you plate 0.1 ml of the second dilution onto each of two plates of MacConkey Agar. After appropriate incubation, you find that one plate contains 155 red colonies and 45 white colonies, and the other plate contains 160 red colonies and 35 white colonies. What was the concentration of gram-negative, lactose-fermenting CFUs per ml of the pond water sample?
As we see in Experiment 4 and Appendix D and throughout the course, only gram-negative bacteria are expected to grow (form colonies) on MacConkey Agar. Of these colonies, the red ones are lactose-fermenters. In this problem, the average number of colonies of lactose-fermenting gram-negative bacteria is 157.5.
| dilutions made | X | amount inoculated | = | "plated dilution" |
| 1/100 X 1/100 | X | 1/10 | = | 1/105 or 10–5 |
|
|
||||
| dilution factor | X | no. colonies | = | no. CFUs/ml |
| 105 | X | 157.5 | = | 1.6 X 107 (rounded off) |
6. One should expect the same number of CFUs in one ml of an undiluted sample as in 10 ml of a 1/10 dilution of the same sample.
If you dilute something so it's 1/10 the concentration, then you will need 10 times as much to get the same number of CFUs. Consider this analogy: To get 10 dollars, you can work 1 hour for 10 dollars an hour or 10 hours for 1 dollar an hour.
7. The same dilution can be obtained in each of the following situations:
a. The addition of 1 ml of a sample to 9 ml of sterile diluent.
b. The addition of 3 ml of the same sample to 27 ml of diluent.
c. The addition of 11 ml of the same sample to 99 ml of diluent.
In each case, you are keeping the same proportions in order to achieve the same concentration. It's like preparing a recipe for a greater or lesser amount of dinner guests.
8. You are given a flask containing a 1/10 dilution of sauerkraut juice. Without making any further dilutions, how much of this dilution of juice can be plated in order to achieve each of the following plated dilutions? (As these will be pour-plates – as opposed to the surface-inoculated plates in problem 1 – you can inoculate more than 0.1 ml to your plates.)
| plated dilution | amount of 1/10 dilution of juice |
| 100 | 10 ml |
| 10–1 | 1 ml |
| 10–2 | 0.1 ml |

9. One gram of yogurt was added to 99 ml of sterile diluent. Decimal (1/10) dilutions were then made, and one-tenth ml was plated in duplicate on Plate Count Agar. After incubation, the following colony counts were made:
| dilution | colony count |
| initial dilution (1/100) | TNTC, TNTC |
| first 1/10 dilution | TNTC, TNTC |
| second 1/10 dilution | 412, 422 |
| third 1/10 dilution | 54, 56 |
| fourth 1/10 dilution | 6, 4 |
Using the above data, calculate the number of CFUs per gram of the yogurt.
| dilutions made | X | amount inoculated | = | "plated dilution" |
| 1/100 X 1/10 X 1/10 X 1/10 | X | 1/10 | = | 1/106 or 10–6 |
|
|
||||
| dilution factor | X | no. colonies | = | no. CFUs/ml |
| 106 | X | 55 | = | 5.5 X 107 |
10. One gram of hamburger was added to a 99 ml dilution blank. Two 1/10 dilutions were then made. From the last dilution made, 0.2 ml was plated onto an all-purpose medium. After incubation, 60 colonies were counted.
Each of the 60 colonies was inoculated onto a slant of Heart Infusion Agar (to grow cultures for gram staining) and into a tube of Glucose Fermentation Broth. Exactly one half of the cultures were determined to be gram-negative. Of these gram-negative cultures, twelve produced acid and gas in the broth and the rest produced only acid.
As indication of acid (with or without gas) indicates fermentation, therefore all of the gram-negative colonies in this problem ferment glucose.
Determine the number of gram-negative, glucose-fermenting CFUs per gram of the meat.
| dilutions made | X | amount inoculated | = | "plated dilution" |
| 1/100 X 1/10 X 1/10 | X | 2/10 | = | 2/105 or 2 X 10–5 (easier to keep it as the fraction) |
|
|
||||
| dilution factor | X | no. colonies | = | no. CFUs/g |
| 105/2 (simple inverse of 2/105) |
X | 30 | = | 15 X 105 or 1.5 X 106 |
|
Page last modified on 2/12/12 at 7:00 PM, CST. |